Electric Current

                                                                        Direct Current Circuit
                                                                              Electric Current
If there is a net flow of charge through a given section of the conductor, then it constitutes an electric current.
If ‘q’ is the magnitude of charge flowing in time ‘t’ second, then
Electric Current(I) = ()/time taken (t)
=    q/t
=    ne/t
The current is a scalar quantity and its unit is Ampere(A) in the SI system.
When q= 1C, and t = 1 Sec, then
I = /
= 1 C/s = 1 A
Thus, Current is said to be 1A if one Coulomb of charge flows in one Second through any section of the
conductor.
                                        Charge Carrier or Current carrier
a)The charged particles which contribute to the electric current are called charge carrier or current carrier. b)For metallic conductor charge carriers are electrons.                                                                                                                  c)In Electrolytes (eg. Solution of CuSo4, Nacl, etc) charge carriers are +ve or –ve charged ions.                                   d)In gases electrons and +vly charged ions is charge carrier.In Semiconductors electrons and holes is charge carrier.
Types of Current
Direct Current
• The current whose magnitude and direction does not change with time.
Alternating Current
• The current whose magnitude changes continuously and direction changes periodically

Current Density
• The current flowing per unit cross-sectional area perpendicular to the direction of flow is called current density. current density (j)=I/A
• It is a vector quantity that has the same direction as the velocity of moving charge if they are +ve and opposite direction if they are –ve (ie. along the direction of current).
                                                #Mechanism of metallic conduction and Drift velocity
                                                               #Mechanism of Metallic Conduction:  In normal state (without electric field ‘E’) electrons in a metallic conductor are moving randomly in all directions so there is no net flow of charge and hence current is zero in the conductor.
When an electric field is applied across this conductor a force starts acting on these charges. Due to this force, all the electrons start to drift in a fixed direction and there exists electric current in the metallic conductor.
This velocity with average value is called drift velocity. All the electrons move with the same drift velocity in the same direction (ie. Opposite to ‘E’).
Drift Velocity :
The average velocity attains by the free electrons in a conductor after the application of the electric field is called drift velocity.
The expression for drift velocity is:
Velocity drift = −**/Ʈ
Where, Ʈ = average collision time, E = electric field, m= mass of electron
The relation between Drift velocity and electric current:
Consider a metallic conductor of length (L) and cross-sectional area ’ A ’ as in the figure. Let n be the number of
free electrons per unit volume and ‘ e ’ be the charge of the electron, then
The volume of the conductor(V) = AL
Total no of electrons in the conductor(N) = n.AL
The total charge on the conductor (Q) = nAL.e
When the battery is connected across the conductor, the electrons start to move in a fixed direction with drift
velocity ( v d ). If total charge ‘Q ’ drift through length ‘L ’ in time ‘t ’, then-current ‘I ’ through the conductor is
Current (I) =/= /)                                                                                                                                                                                                        = neA*(/)                                                                                                                                                                                                              = neA . V d   [ where L/t = V d : drift velocity of electron⌉
This is the required relation between drift velocity and electric current
Again,
Current density (J) = ()−()= /= n e v d
Current density(J) = n e v d
This is the relation between drift velocity and current density.
                                                                                   Ohm’s Law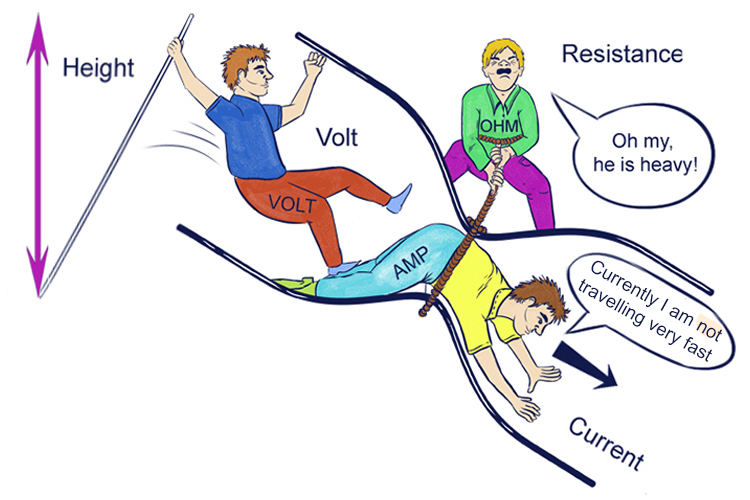
Statement:
The electrical current passing through a conductor is directly proportional to the potential difference across its end under constant physical condition ( tempr , mechanical strain.etc)
If ‘I’ is current, ‘V’ is the potential difference between two ends, then,
I α V
I = C. V ;
where ‘C’ is proportionality constant & its value is C=1
I =1.V
V = I.R
Where R is the resistance of the conductor.
 Experimental verification of Ohm’s Law:Fig: Figure shows an experimental setup to verify Ohm’s law. It consists of a resistor ‘R’ connected in series with a battery, ammeter, and rheostat through a one-way key(k). A voltmeter ‘V’ is connected across ‘R’ to measure the p.d. across it.
By adjusting the rheostat the current in the circuit can be varied and the corresponding value of current and voltage is noted. When the graph is plotted between ‘I’ and ‘V’, then a straight line passing through the origin is obtained.
This straight line passing through the origin shows that current through the conductor is directly proportional to the p.d across it. i.e. I α V
Ohmic and non-ohmic Conductor.
• Ohmic conductor: Conductors that obey Ohm’s law are called an ohmic conductor. eg. Most of the metallic conductors are the example of an ohmic conductor.
• Non-Ohmic Conductor: Which does not obey Ohm’s law. Eg. Semiconductor diodes.
Fig:
                                 Electrical Resistance(R)
• The property of the conductor which oppose the flow of charge is called electrical
resistance.
• The collision of the electrons with atoms or ions is the main cause of resistance. So resistance depends on
The nature of the material of the wire or conductor.
The dimension of the wire i.e. length, the thickness of the wire.
It depends on the temperature of the material.
Temperature Coefficient of resistance(α)
•The resistance of the conductor is observed to increase with the increase in temperature. If R 1 and R 2 be the resistance of the conductor at temperature θ 1 and θ 2,0 C, then increase in resistance (R 2 -R 1 ) is directly proportional to the initial resistance R 1
and rise in temp r (θ 2 -θ 1 ), then i.e.( R2 -R 1 ) α R 1 (θ 2- θ 1)
( R2 -R 1 ) = α R 1( θ 2 -θ 1)
Where α is the proportionality constant called the temperature coefficient of resistance
R2 = R 1 [1+ α* Δθ]
=α(R2−R1)/R1.Δθ
*Metals have + Ve temp r coeff . of resistance.
*Semiconductors have Ve temp r coefficient of resistance
*Material like manganian, constantan, etc have nearly zero tempr coeff  of resistance
*Temp r Coeff . is Ve for electrolytes, carbon, mica, etc
                                               Specific resistance or Resistivity(ρ)
•It is observed that
Resistance(R) α length(l) ………. (1)
Resistance(R)  α   1/Cross−sectionalarea(A)……… (2)
Combining (1) and (2), R α  /
Where ρ is the proportionality constant called resistivity whose value depends on the nature of the material.
when l =1m and A = 1 m2, then
R = ρ
Thus,
the resistivity of a material is the resistance offered by the material having a unit length and unit
cross-sectional area. Its unit is Ω m in the SI system.
                   The relation between current density(J) and Electric field intensity(E)
We have,
Current Density(J)=/
=(/)*(/)
=/*/()
=/*(/)
J = σ E
                                             Combination of Resistors
1. Series Combination of Resistors
When two or more resistors are connected end to end so that current through each resistor is same then such combination is called Series combination of resistors. Such a combination is preferred when high resistance is required in the circuit.
Consider three resistors R1, R2, and R3 are connected in series with a battery of voltage ‘V’ as in fig. In such a combination current ‘I’ is the same through each resistor. Let V 1, V 2, and V 3 be the p.d across the resistor R 1, R 2, and R 3 respectively, then The p.d. across the entire combination is the sum of individual p.d.
i.e. V = V1 +V 2 +V 3
          = I.R1 + I.R 2 +I.R 3
           = I . (R1 +R 2 +R 3
/= R 1 +R 2 +R 3                           [= Rs equivalent resistance in series combination]
In general, if there are ‘ n’ number of resistors connected in series then their equivalent resistance will be
Rs = R 1 +R 2 +R 3
Rs = R 1 + R 2 + ………….. + R n
Characteristics of Series Combination of Resistors:
The current through each resistor is the same.
Total p.d. is the sum of individual p.d.
The equivalent resistance is greater than the greatest individual resistance.
The equivalent resistance is equal to the sum of individual resistance.
2. Parallel Combination of resistors
When two or more resistors are connected between the same two points so that p.d across each the resistor is the same then such combination is called Series combination of resistors.
Consider three resistors R1 , R 2, and R 3 are connected in parallel between two points A and B. when a battery of voltage ‘V’ is connected across this combination, p.d. across each resistor is the same but the current is different. Let ‘V’ be the p.d. across A and B and I 1, I 2 and I 3 be the current through resistor R 1, R 2 and R 3 respectively, then
The total current ‘I’ is divided into I 1, I 2, and I 3 so
I = I 1 + I 2 + I 3 ………….. (1)
From Ohm’s Law,                                                                 
I1 .R 1 = V AB =V
I1 =/
Similarly,
I2 =/ and I 3 =/
Using the value of I1, I 2 and I 3 in eq. (1) then
I = I1 +I 2 +I 3                                                                                                                                                                                                               =/+/+/                                                                                                                                                                                          =/+/+/
/=/+/+/
If there are ‘n’ number of resistors connected in parallel, then their equivalent resistance will be
/=/+/+/
/=/+/+………………. +/
Characteristics of Parallel Combination of Resistors:
P.d. across each resistor is the same.
Total current is the sum of individual current.
Effective resistance is less than the smallest resistance in the combination.